Next: Spectrum evaluation for perturbed
Up: Microresonators
Previous: Shifting the cavity between
Contents
Tuning
From a practical point of view, the ability to tune the spectral response of
the resonators is an essential feature. As mentioned in
Section 1.4.5, a tuning mechanism can relax otherwise
quite demanding fabrication tolerances, and it can also help to eliminate any
unwanted temperature induced deviations of the spectral response.
For the applications of microresonators as tunable wavelength filters,
suitable materials are introduced that permit to change slightly the
refractive index of the cavity core by external mechanisms like electro- or
thermo-optic effects. Then using Eq. (1.21), shifts in resonance
wavelengths due to changes of the core refractive index are given by
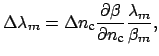 |
(4.15) |
where the derivative of the phase propagation constant with respect to the core
refractive index can be approximated by Eq. (2.31) as
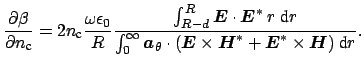 |
(4.16) |
Here
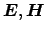 are the electric field and magnetic field of the cavity
mode associated with the
are the electric field and magnetic field of the cavity
mode associated with the  'th order resonance.
'th order resonance.
Subsections




Next: Spectrum evaluation for perturbed
Up: Microresonators
Previous: Shifting the cavity between
Contents
Kirankumar Hiremath
2005-09-23