Shifting the cavity between fixed bus waveguides

From the view point of realization of microresonators, it is useful to understand the effects of a displacement of the cavity with respect to the adjacent straight waveguides. This means, studying the effects of changing both separation distances, while keeping $g + \tilde{g}$ = constant. Figure 4.18 shows corresponding simulation results for the previous ring resonator.

Figure: Spectral response of the ring resonator for shifting the cavity between fixed straight waveguides. For $g + \tilde{g}$ = $0.4 \,\mu$m, the plots show the through power (first row) and the drop power (second row) for ring resonators with $g=0.10 \,\mu$m and $\tilde{g} = 0.30 \,\mu$m (dash-dotted line), $g=0.15 \,\mu$m and $\tilde{g} = 0.25 \,\mu$m (dashed line), $g=0.20 \,\mu$m and $\tilde{g} = 0.20 \,\mu$m (solid line).

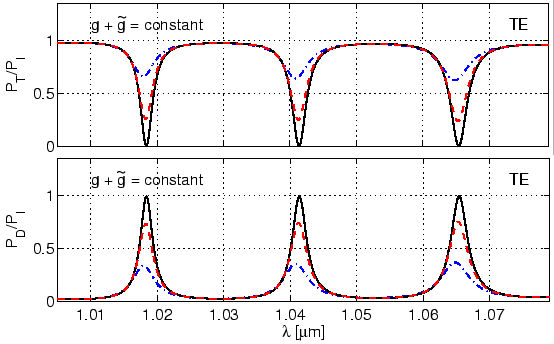
While for the off-resonance wavelengths there are hardly any differences, close to the resonance wavelengths, one can observe substantial changes. As seen from the right plot of Figure 4.16, for growing $g$ with $g < \tilde{g}$, the through power steadily decreases and the drop power steadily increases (also see Figure 4.18). As discussed before, for $g=\tilde{g}$, at resonance there is complete transfer of the input power to the drop port. For larger $g$ with $g > \tilde{g}$, the reverse effect takes place, i.e. the through power steadily increases, and the drop power steadily decreases.

For constant $g + \tilde{g}$, the simulations of Figure 4.18 systematically investigate the consequence of shifting the cavity closer to the lower straight waveguide. It will be equally interesting to investigate the effect of shifting the cavity closer to the upper (input) waveguide. For this, it is sufficient to know the influence of interchanging the separation distances $g$ and $\tilde{g}$ on the spectral response.

Figure: Power transmissions of the ring resonator for interchanging $g$ and $\tilde{g}$. For $g + \tilde{g} = 0.4 \,\mu$m, the plots show the through power (top plot) and the dropped power (middle plot) for $g=0.25 \,\mu$m, $\tilde{g}=0.15 \,\mu$m (dashed lines) and $g=0.15 \,\mu$m, $\tilde{g} = 0.25 \,\mu$m (solid lines), whereas the bottom plot shows the difference between the respective through powers (dash-dotted line), drop powers (circles) for the two settings. Note that the curves for $P_{\mbox{\scriptsize D}}$ and -on the scale of the figure- also the curves for $P_{\mbox{\scriptsize T}}$ are superimposed.

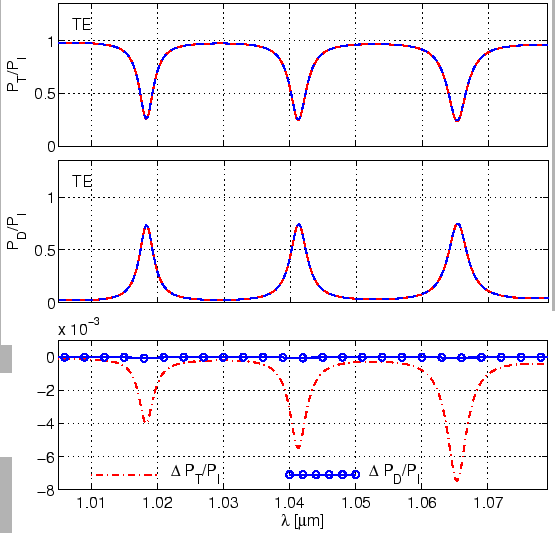As an illustration, Figure 4.19 compares the ring resonator spectral response for $g=0.25 \,\mu$m, $\tilde{g}=0.15 \,\mu$m and $g=0.15 \,\mu$m, $\tilde{g} = 0.25 \,\mu$m. On the scale of the figure, the curves (solid line and dashed line) for the through power (first row) and the dropped power (second row) are almost indistinguishable. But when the difference between the two results is plotted (third row), one sees that power through for the setting $g=0.25 \,\mu$m, $\tilde{g}=0.15 \,\mu$m is more than that for $g=0.15 \,\mu$m, $\tilde{g} = 0.25 \,\mu$m, whereas the drop power is equal in both cases. The same behaviour is also observed in case of corresponding simulations for the disk resonator, which are shown in Figure 4.20. In that case, one can clearly distinguish the two through power curves around the resonances of the TE$_{1}$ mode. But in both cases, the dropped power remains unchanged.

Figure: Power transmissions of the disk resonator for interchanging $g$ and $\tilde{g}$ with $g + \tilde{g} = 0.4 \,\mu$m. The interpretation of the curves is the same as for Figure 4.19.

The invariance of the drop power for interchange of $g$ and $\tilde{g}$ can be explained by flipping the resonator along the $z$ axis, and using $\cal{S}_{\tilde{\mbox{\scriptsize B}}\mbox{\scriptsize A}} = \cal{S}_{\mbox{\scriptsize A} \tilde{\mbox{\scriptsize B}}}$ from Eq. (4.6).

This can also be explained by using Eq. (4.9) . For the resonator setting as in Figure 4.1 (symmetrical around the plane $z=0$), interchanging the separation distances $g$ and $\tilde{g}$ is equivalent to changing the setting with input at port A to a setting, where port $\tilde{\mbox{A}}$ is excited.

According to Eq. (4.9), the power at port $\tilde{\mbox{B}}$ for $P_{\mbox{\scriptsize I}} = 1$ and $P_{\mbox{\scriptsize A}} = 0$, which is the drop power with $g=0.25 \,\mu$m, $\tilde{g}=0.15 \,\mu$m, is the same as the power at port B for $P_{\mbox{\scriptsize I}} = 0$ and $P_{\mbox{\scriptsize A}} = 1$, which is the drop power with $g=0.15 \,\mu$m, $\tilde{g} = 0.25 \,\mu$m. Hence the difference between the drop powers for the two settings (depicted by a line with circles in the bottom plot of Figures 4.19, 4.20) is zero.

What concerns the nonnegligible difference in the throughput power (dash-dotted line in the last plots of Figure 4.19, 4.20, one can consider the ``ideal'' resonator discussed in context of Eq. (4.12). If the attenuation corresponding to the propagation along the cavity is negligible, i.e. $\alpha \approx 0$ for the cavity mode, and if the coupling is lossless, then for monomodal port waveguides, one has

$$\vert{\cal{S}}_{\mbox{\scriptsize B} \mbox{\scriptsize A}}\vert^2 + \vert{\cal{S}}_{\tilde{\mbox{\scriptsize B}} \mbox{\scriptsize A}}\vert^2 = 1, P_{\mbox{\scriptsize I}} = 1, P_{\mbox{\scriptsize A}} = 0,$$ (4.13)

$$\vert{\cal{S}}_{\tilde{\mbox{\scriptsize B}} \tilde{\mbox{\script... ...t^2 + \vert{\cal{S}}_{\mbox{\scriptsize B} \tilde{\mbox{\scriptsize A}}}\vert^2 = 1, P_{\mbox{\scriptsize A}} = 1, P_{\mbox{\scriptsize I}} = 0.$$ (4.14)

From the Eq. (4.9), one has ${\cal{S}}_{\tilde{\mbox{\scriptsize B}} \mbox{\scriptsize A}} = {\cal{S}}_{\mbox{\scriptsize B} \tilde{\mbox{\scriptsize A}}}$, which leads to $\vert{\cal{S}}_{\mbox{\scriptsize B} \mbox{\scriptsize A}}\vert^2 =\vert{\cal{S}}_{\tilde{\mbox{\scriptsize B}} \tilde{\mbox{\scriptsize A}}}\vert^2$, i.e. for the low loss resonator, given input only at port A, the output power at port B is exactly the same as the output power at port $\tilde{\mbox{B}}$ for input given only at port $\tilde{\mbox{A}}$. Or in the other words, interchanging $g$ and $\tilde{g}$ does not affect the through power.

For the ring resonator in Figure 4.19, such an ``ideal'' situation is realized (see Figure 3.2, a well guided cavity mode, and Figure 3.4, almost lossless coupling). Therefore the difference in the throughput power for these simulations is quite small. As the wavelength increases, the attenuation of the cavity mode increases, resulting in corresponding growing deviation $\Delta P_{\mbox{\scriptsize T}}/P_{\mbox{\scriptsize I}}$. This can be clearly seen for the resonances of the TE$_{0}$ mode.

The simulations of the disk resonator in Figure 4.20, involve a substantially lossy TE$_{1}$ whispering gallery mode (see Figure 3.8). Here $\vert{\cal{S}}_{\mbox{\scriptsize B} \mbox{\scriptsize A}}\vert^2 \neq \vert{\cal{S}}_{\tilde{\mbox{\scriptsize B}} \tilde{\mbox{\scriptsize A}}}\vert^2$, which is evident from the significant difference of the through power $P_{\mbox{\scriptsize T}}$. Apart from the minor differences near the resonances of the TE$_{0}$ mode, one can see the pronounced deviations near the resonances of the TE$_{1}$ mode.

It should be emphasized that the invariance of the dropped power for interchanging the gaps can be used as an additional check of the consistency of the model. The almost perfect agreement of the the curves for the dropped power in Figures 4.19 and 4.20 shows that the present CMT based model of microresonators satisfies this constraint very well.